How To Find Area Of A Base

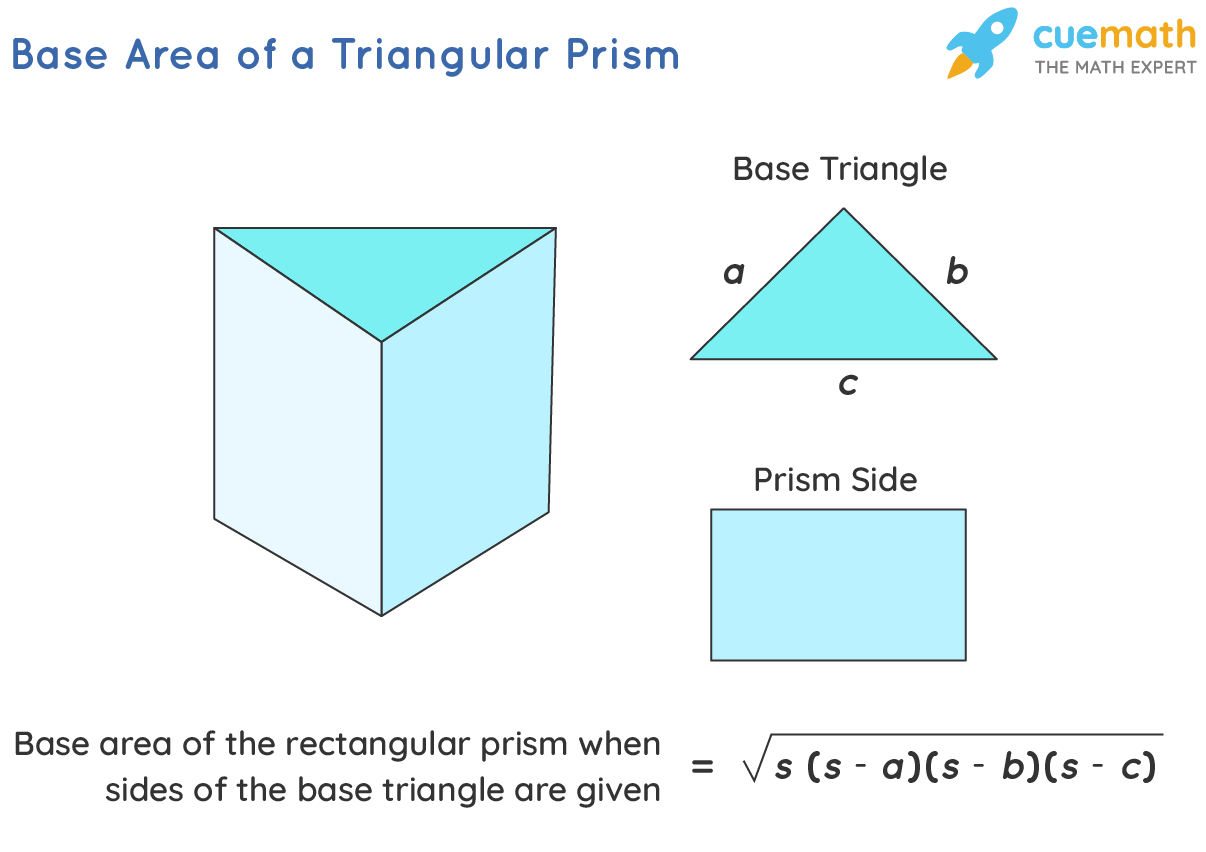
Base Area of a Triangular Prism

In this department, we will discuss the base expanse of a triangular prism along with solved examples. The base of operations area of a triangular prism is the area of the triangle of the prism. Allow usa starting time with the pre-required noesis to sympathize the topic base of operations expanse of a triangular prism. Recall that a prism is a solid which has identical polygon ends (bases), flat parallelogram sides, and has the same cross-department all along its length.

A prism having 3 faces as rectangles and two triangles equally its bases (one triangle acts equally the elevation) is called a triangular prism. It's a three-sided prism where the base and tiptop are equal triangles and the remaining 3 sides are rectangles. Both of the triangles are parallel and congruent to each other. The rectangles need not be congruent with each other.

1.	What Is Base Area of a Triangular Prism?
2.	Base Expanse of a Triangular Prism Using Sides
3.	Base Area of a Triangular Prism Formula with Tiptop and Base
4.	FAQs on Base of operations Area of Triangular Prism

What Is Base Expanse of a Triangular Prism?

The base expanse of a triangular prism is divers as the full area covered by the bases of a triangular prism. The base of operations area of the triangular prism is expressed in square units. The area of the base of a triangular prism can exist calculated using dissimilar formulas, depending upon the known parameters and type of triangle in the base of operations. Let the states understand these formulas in the post-obit sections.

Base Area of a Triangular Prism if the Sides of Its Triangle Are Given

For a triangular prism, if the length of the sides of the triangular base of the prism is given, and so its base of operations area can exist given by:
Base expanse of a triangular prism = Area of the base triangle

Using Heron's formula for the area of a triangle nosotros go,
Base area of a triangular prism = √[south(s − a)(s − b)(southward − c)]
where,

a, b, c are the lengths of the sides of the base triangle, and,
due south is its semi-perimeter and is equal to (a + b + c)/ii

base area of triangular prism

Example: How to notice the base area of a triangular prism given the length of the sides of the base triangle: a = twenty units, b = x units, and c = xx units?

Semi-perimeter (s) = (20 + 10 + 20)/2 = 25 units

Base area of the given triangular prism = √[s(s − a)(south − b)(south − c)] = √[25(25 − 20)(25 − 10)(25 − twenty)] = √[25(5)(15)(5)] = 25√15 square units.

Base Surface area of a Triangular Prism Formula if the Base of operations and Top of the Triangle Are Given

For a triangular prism, if the length of the base and height of the base triangle is given, and then its base area can exist given by,

Base area of a triangular prism = Area of the base triangle

Base surface area of a triangular prism = (ane/2) × b × h

where,

b is the length of the base of operations of the triangle, and,
h is the peak of the triangle

base area of triangular prism

Example: How to discover the base area of a triangular prism given the height of the triangle h = x units and base b = 20 units?

Solution: Base expanse of the given triangular prism = (one/two) × b × h = (1/2) × 20 × ten = 100 square units

Important Notes on Base of operations Expanse of a Triangular Prism

The base of operations expanse of a triangular prism can be calculated when three sides of the base triangle are given.
The base expanse of a triangular prism can be calculated when the base length and height of the base of operations triangle are given.

Related Topics on Base Surface area of a Triangular Prism

Triangular Prism
Volume of Square Prism
Surface Surface area of Prism

Solved Examples on Base of operations Area of a Triangular Prism

Example ane: Detect the base expanse of the triangular prism whose base of operations triangle has the meridian = lx units and base length = 40 units.

Solution:

Meridian of the triangular prism = lx units

Base length of the triangular prism = xl units

Base area of the given triangular prism = 1/2 × Base length × Height of the base triangle = 1/two × 60 × 40 = 120 square units

Respond: Base area of the given triangular prism = 120 foursquare units
Instance ii: Find the base area of the triangular prism whose base triangle has the length of the sides a= 3 units, b = four units, and c = v units.

Solution:

Length of the sides a= 3 units, b = 4 units, and c = 5 units.

Semi-perimeter of the base of operations triangle (south) = (3 + four + 5)/2 = half dozen units

Base area of the given triangular prism = √[due south(south−a)(southward−b)(s−c)]due south = √[6(6−3)(half-dozen−4)(6−v)] = √[6(3)(2)(1)] = 6 foursquare units

Reply: Base expanse of the given triangular prism = vi square units.

go to slidego to slide

Breakup tough concepts through elementary visuals.

Math will no longer exist a tough subject field, particularly when you understand the concepts through visualizations.

Book a Free Trial Form

Exercise Questions on Base Area of a Triangular Prism

go to slidego to slide

FAQs on the Base Expanse of a Triangular Prism

What Is Base of operations Surface area Triangular Prism?

The base surface area of a triangular prism is divers equally the region or area covered past the bases of a triangular prism. A triangular prism has 2 triangle-shaped bases.

How to Calculate the Base Area of a Triangular Prism?

The base area of a triangular prism tin can be calculated equally,

Note downward the dimensions of the triangle in bases.
Apply the formulas to calculate the area of the base of operations triangle and multiply it by two.
Express the result with a suitable unit.

What Are the Formulas to Find Base of operations Area of a Triangular Prism?

The formulas that tin be used to find the base area of a triangular prism are,

When base of operations triangle is equilateral, with each side 'a', so surface area = √3aⁱⁱ/4.
When base triangle's base 'b' and summit 'h' is given, then area = (1/2) bh.
When base triangle is a right-angled triangle, with 2 legs 'b' and 'h' so area = (one/2) bh.
When base triangle is an isosceles triangle with its sides to be 'a', 'a', and 'b' so its area is (b/four) · √(4a² - b²)
When base triangle is scalene, with all 3 sides 'a', 'b', and 'c' are given, so area = √[due south(due south - a)(s - b)(s - c)], where southward = (a + b + c)/2.
When base of operations triangle'due south 2 sides 'a' and 'b' and the included bending 'θ' are given, then area = i/2 ab sin θ.

What Are the Units Used to Limited Base Area of a Triangular Prism?

The units used to express the base area of a triangular prism are in^two, m², cm², ft², etc. It is expressed in square units.

How to Calculate the Base of operations Length Using Base of operations Expanse of a Triangular Prism?

Using the expanse of triangle formula for the given type of triangle in base, we can summate the base of operations length past substituting the known values in the formula and solve.